A Different Approach, A Better Result.
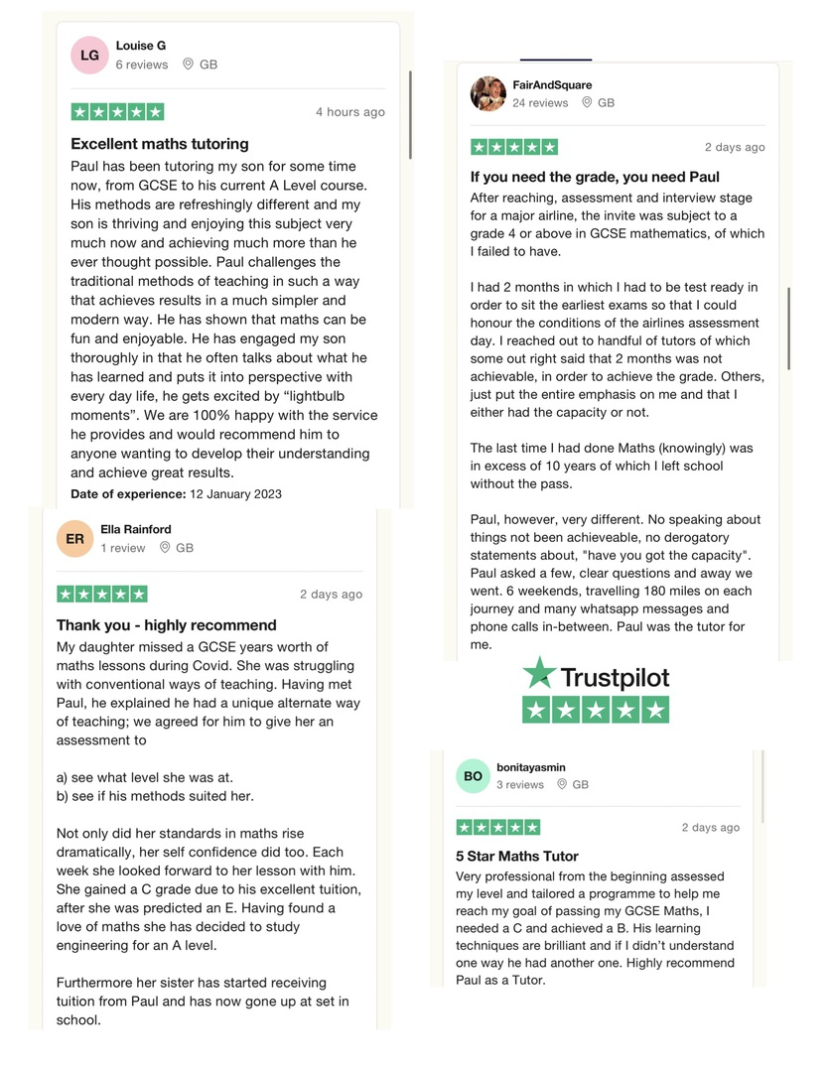
"I was amazed at what I could do with this method..." Online reviewer
One-on-one maths help for:
GCSE,
Functional Skills Level 2
IGCSE,
GCSE Further Maths
A-Level,
IB,
A-Level Further Maths,
University
or enjoyment.
Choose between weekly lessons or intensive courses tailored to your schedule.
Highlights include: a 98% success rate, flexible online or face-to-face tuition, a highly qualified and experienced maths communicator, recommended by parents and students, easier methods approved by Edexcel exam board, DBS checked, and expertise across all exam boards.
A Video Example of One of My Methods
Unlocking the beauty of mathematics...more than simply maths tuition
My Philosophy and Mission Statement
With over 23 years of experience of unlocking the apparent complexity of mathematics, I have constantly sought to be passionate about the subject I teach. I am dedicated to helping students understand and excel in maths, going beyond the role of a tutor.
Apart from presenting an alternate view of maths, one of my greatest strengths lies in my ability to quickly identify students' problem areas, and become attuned to what they understand. This allows me to provide targeted support and guidance, helping them regain their confidence. I take great pride in helping students achieve grades that surpass their expectations, proving that they are capable of more than they may have realized.
Moreover, I have a knack for recognizing students who possess exceptional mathematical abilities, often overlooked by the rigid, one-size-fits-all structure of traditional schooling. By identifying these hidden talents, I facilitate their development and help them reach their full potential.
As a result of these academic accomplishments, this empowers students to reclaim their self-respect. Through my guidance, students not only improve their math skills but also develop a strong sense of self-worth and confidence. Witnessing this transformation is immensely gratifying, both for the parents of students and for me as an educator.
Above all, the feedback I receive from students is a testament to the impact the method has on their lives. Many students express that they begin to enjoy mathematics again. Even more remarkably, my lessons generate a sense of excitement.. I strive to create an engaging learning environment where students feel motivated and inspired to explore this fascinating world. Recently I have submitted my methods to Edexcel exam board and they have been approved for use in exams. A personal triumph.
In summary, my extensive experience, passion for mathematics, ability to identify and address students' needs, and commitment to fostering a positive learning environment have enabled me to make a significant difference to my students. I am dedicated to not only helping them excel academically but also reigniting their love for maths.
Typically, private tutors follow the same teaching methods as schools. However, if the traditional school approach has proven ineffective for you or your child, it's clear that more of the same won't yield positive results. That's why I offer a completely different way of teaching. None of the approaches I employ resemble the conventional school methods, so please keep that in mind when considering my services. Unfortunately, the evidence shows that these conventional methods often fall short, and your decision to seek additional help serves as further proof of their limitations.
Driven by my frustration with the way mathematics is generally taught in schools, I have developed a new and innovative approach based on intuition rather than rote memorization, on understanding rather than blind acceptance, on problem-solving skills rather than getting stuck and feeling lost, and on fostering confidence and enjoyment rather than shame and dislike.
Here are some of the key features and innovations of my teaching method (and now approved by Edexcel):
1. An easier, highly efficient, intuitive and enjoyable approach to multiplying numbers, applicable across 11 different areas of mathematics, from 2 digit (41 x 32) up to any number of digits (e.g. 1701 x 3212)
2. Three simple rules that serve as a thread that when pulled, reveals the tapestry of mathematics.
3. Clear comprehension of the purpose of algebra (which school rarely explains, and students rarely know it even has a purpose).
4. Effortless techniques for squaring, cubing, and finding higher powers of any number.
5. Utilisation of a one single concept to understand indices, decimal multiplication, area calculations, standard form, and more.
6. Subliminal teaching of algebra and (and even calculus), not only making them easier to comprehend, but giving an insight into finding 4 ways to find a gradient on a quadratic graph - a perspective I've never seen in any textbook.
7. Apart from an alternative quadratic formula which reveals more information than the standard one in general use, but a way to solve quadratics without the use of any algebra in a few seconds.
8. Extremely fast mental or quick paper calculations of percentages.
9. Unique explanation of trigonometry, shedding a new light on its principles and applications.
10. Employment of an advanced yet straightforward method to find the highest common factor (HCF), lowest common multiple (LCM), and other free information in one step.
11. Genuinely understand area and volume and their correct definition.
[yes, it goes up to 11 - there are more than this, but felt appropriate to stop here!]
For the A-Level syllabus, in addition to the aforementioned points, my course includes:
1. Deep understanding of differentiation and integration, initially explained through numbers and visual aids only.
2. Comprehensive insight into the purpose and quick solutions for quadratic and cubic equations, including techniques to forever avoid algebraic division.
3. Mastery of the binomial theorem using a single method, recognition of its hidden applications in various mathematical aspects, rapid computation of higher powers and binomial expansions compared to traditional methods, and an entirely new and straightforward approach to determine any line of Pascal's triangle.
4. More expanded utilisation of Pythagoras' theorem and its uses beyond triangles.
5. Understanding the factors that make quadratics "factorisable" and the ones that are not, along with how common they are.
6. Uncovering the secret of algebra's true nature to more complicated forms.
7. Comprehending the outcome when combining sine and cosine waves.
8. Understanding the origin of the derivation of SUVAT equations.
9. Exploring the reasoning behind solving quadratics in projectile problems.
10. Discovering where the quadratic formula comes from.
11. Understanding the purpose of binomial expansion.
12. Discovering the origin of why A = πr2 and why C= 2πr, with easier ways to find arc lengths and sector areas.
With my teaching method, anxiety and fear are eliminated. I have vast experience working with students who have lost their confidence, struggle to understand their teacher's explanations, can solve problems in class but falter during exams, feel lost and anticipate failure, dislike maths and find it uninteresting, or experience anxiety when confronted with mathematical concepts. If you or your child fall into any of these categories, I can provide the necessary assistance.
While the aforementioned reasons are frequently why students seek my services, I am also equipped to help advanced students by challenging their understanding and introducing novel perspectives. It is striking with advanced students just how much my method fits into their pattern of thinking like a jigsaw piece, enable them to accelerate rapidly through content, concepts and anticipating what will follow.
This approach has resulted in a high success rate among my students, with a 100% success rate achieved in 2013 and 2017, and typically only one student falling slightly short of their desired outcome. This success rate surpasses that of schools, which cater to a wide range of students. Most of my students come to me because they are struggling due to the limitations of the school system.
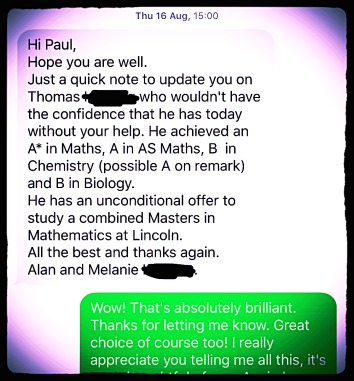
ABOVE: I was extremely gratified and pleased to receive this message after the GCSE results of 2016.
(See below for update in 2018!)
Even more about my method...!
As above, over 23 years, I have developed my own unique method for teaching mathematics, keeping the psychology of the learner in mind. It continues to evolve for efficiency, understanding, pattern and fluency. It incorporates a holistic approach, utilizing fewer methods more frequently, which reduces the burden of learning and fosters this fluency. Unlike traditional school approaches that often resort to saying "it just is" or "you don't need to know that," I strive to explain the "why" behind every concept, placing it in a relevant context. Understanding the reasoning behind mathematical principles enhances the learning experience.
I am proud to share that my method has gained recognition and has been published by the UK's largest publisher, available in both print and Kindle editions on Amazon. I receive messages from individuals worldwide, including countries like India, Argentina, Zimbabwe, Australia, and China, expressing how my approach has finally provided them the breakthrough they needed after struggling for years. Moreover, I have created an online video channel where students can revise the methods as many times as necessary, ensuring continuous support.
What brings me the greatest joy in my work is witnessing the moment when a student comprehends something they once believed was beyond their grasp. Within a safe, secure, and positive learning environment, students achieve remarkable results, often leaving their parents astonished. It is a privilege to demonstrate to students the wonder of mathematics and to reveal its inherent simplicity. The transformation experienced by my students is truly mind-blowing.
One of the most rewarding aspects of my teaching method is that it not only enhances mathematical proficiency, regardless of the starting point, but also allows students to accomplish tasks well beyond their expected abilities. Simultaneously, it helps them shed any feelings of shame and reclaim their self-worth. As a result, parents frequently recommend my services, and I can provide over 100 glowing references from satisfied clients.
I am deeply passionate about learning, and I strive to instill that same passion in my students. My motto, "Get ready... for anything," reflects my goal of equipping students with logical thinking skills that empower them to tackle any question they may encounter. Additionally, I constantly engage in my own learning endeavours to maintain a sense of what it's like to be a student and to prepare myself for any challenge. For instance, I recently completed a second Engineering degree with the Open University, pursued an aircraft technician's course, embarked on learning Polish and even undertook advanced motorcycle riding lessons. Currently I am taking a watch technician's course and I am about to learn how to be a pilot. I also learn more advanced mathematics with a view to gain higher qualifications.
In addition to regular lessons, I offer specialized programs such as a 7-day intensive GCSE course and a 14-day intensive A-level course. These customized packages have proven highly effective, with every student achieving their desired grades. The location is flexible, and I provide all the necessary materials. I am so confident in the results that I guarantee the requested grade. For more details, please see Intensive Courses.
While this brief introduction offers a glimpse into who I am, the best way to learn more is by contacting me directly. Through a discussion about your requirements, I am confident that you will be willing to give my approach a try. Please reach out to me using the contact form or by phone, and I will respond as promptly as possible.
Paul Carson BSc BEng (Hons) AMIMA
Selection of Qualifications
Bachelor of Engineering - BEng (Hons) (Open) 2020
BSc Pure Science (University of St Andrews) - 2002
A at A-Level Maths
A* at GCSE Maths
Level 3 Award in Teaching and Education - 2016
NVQ in Aeronautical Engineering
Current Projects:
Postgraduate Certificate in Space Science (end 2024) Diary here.
Learning the Polish and Irish languages and how to fly a paramotor (although not at the same time).
M820 and T229 modules at the Open University, in Calculus of Variations, Advanced Calculus and Mechanical Engineering: Heat and Flow.
Tutoring Experience:
24 years as a private tutor of all ages from 10 upwards with some school experience in Lincolnshire. I estimate I have taught approximately 10,000 hours in that time.
3 years as an Associate Lecturer with the Open University in Maths and Engineering. I am responsible for 60 adult students per year.
Our Initial Meeting...
My approach is to first identify any issues the student has via an assessment. From this I can develop a strategy for the student to understand maths more easily, which they can take on board to increase in confidence, knowledge and ability. The student will begin to find maths much more easy than they had previously thought. The normal pattern is that the student has failed by using school techniques, so repeating more of the same won't work! My methods are logical, easy and intuitive.